A constitutive Equation for Thermoviscoelastic Behavior of Polymeric Materials
Suri GaneriwalaSpectraQuest Inc., 8227 Hermitage Road, Richmond, VA 23228
Published: October, 01 2004
Abstract
With increasing uses of polymeric materials for providing viscoelastic damping in many mechanical systems and structures, it has become necessary to develop appropriate constitutive equations. Conventional constitutive theories and master curves do not provide a sufficient representation of the linear thermoviscoelastic properties needed for a sound engineering analysis and design. this paper presents a new scheme that yields a complete constitutive equation suitable for analysis of complex problems. the model is based on postulates of generally observed temperature and frequency (or time) dependent behavior of polymeric materials and our recent development of a new model of the glass transition phenomenon. An important part of the derivation is a new description of the glass transition behavior of polymers. The model is internally consistent and makes remarkable predictions. the time-temperature principle is an integral part of this model. An initial test of the new approach was carried out with two different classes of polymers with good results. It is adaptable to most computer systems.
Introduction
Viscoelastic material are valuable for isolation and control of sound and vibration because of their ability to dissipate energy. the damping ability of these materials is increasingly utilized to develop advanced composite materials such as high strength low weight aircraft structures and other engineering components. A distinguishing feature of viscoelastic materials is strong dependence of mechanical properties on temperature and frequency (or time). For an optimum design, dynamic analysis of viscoelastically damped structures must be performed at a design phase. Such engineering analysis requires a constitutive equation for the material viscoelastic behavior.
A Coupled theory of thermoelasticity which includes the effects of both temperature as well as strain histories on the mechanical material functions is not well developed yet. Furthermore, a constitutive theory provides a general form of material functions. the exact constitutive equation has to be derived from studies of mechanical properties over wide ranges of frequency (or time) and temperature. many dynamic mechanical property studies have been reported but appropriate constitutive equations defined over a wide ranges of temperature and frequency have been elusive.
The mechanical behavior of most polymers and amorphous materials can be adequately represented by the theory of viscoelasticity. A broad classes of materials form a disordered solid structure when cooled from the molten state; polymers are just a one class from the list. But because of wide uses of polymers, viscoelasticity is generally associated with polymers only. In This paper, Polymers and polymeric materials will be synchronously used to designate viscoelastic materials.
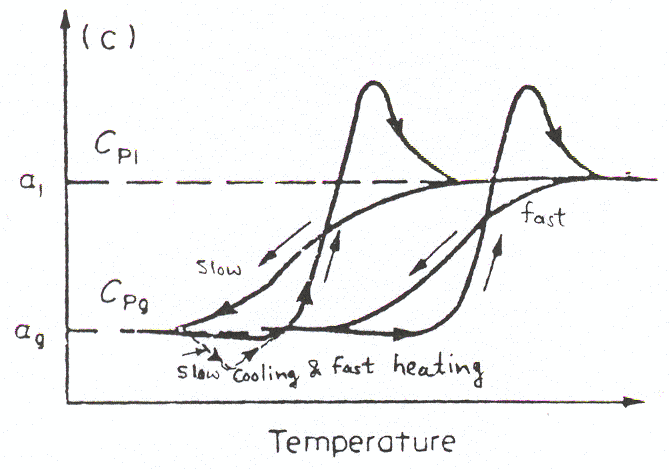
Fig 1: Temperature dependence of properties of glassy materials. notice rate effect, asymmetry, nonlinearity, and hysterisis.